Un viaje alrededor de alfa y omega para estimar la fiabilidad de consistencia interna
Agencias de apoyo
- Dirección General de Investigación y Gestión del Plan Nacional de I D i
- del Ministerio de Economía y Competitividad
- Agencia de Gestión de Ayudas Universitarias y de Investigación AGAUR de la Generalitat de Catalunya
Resumen
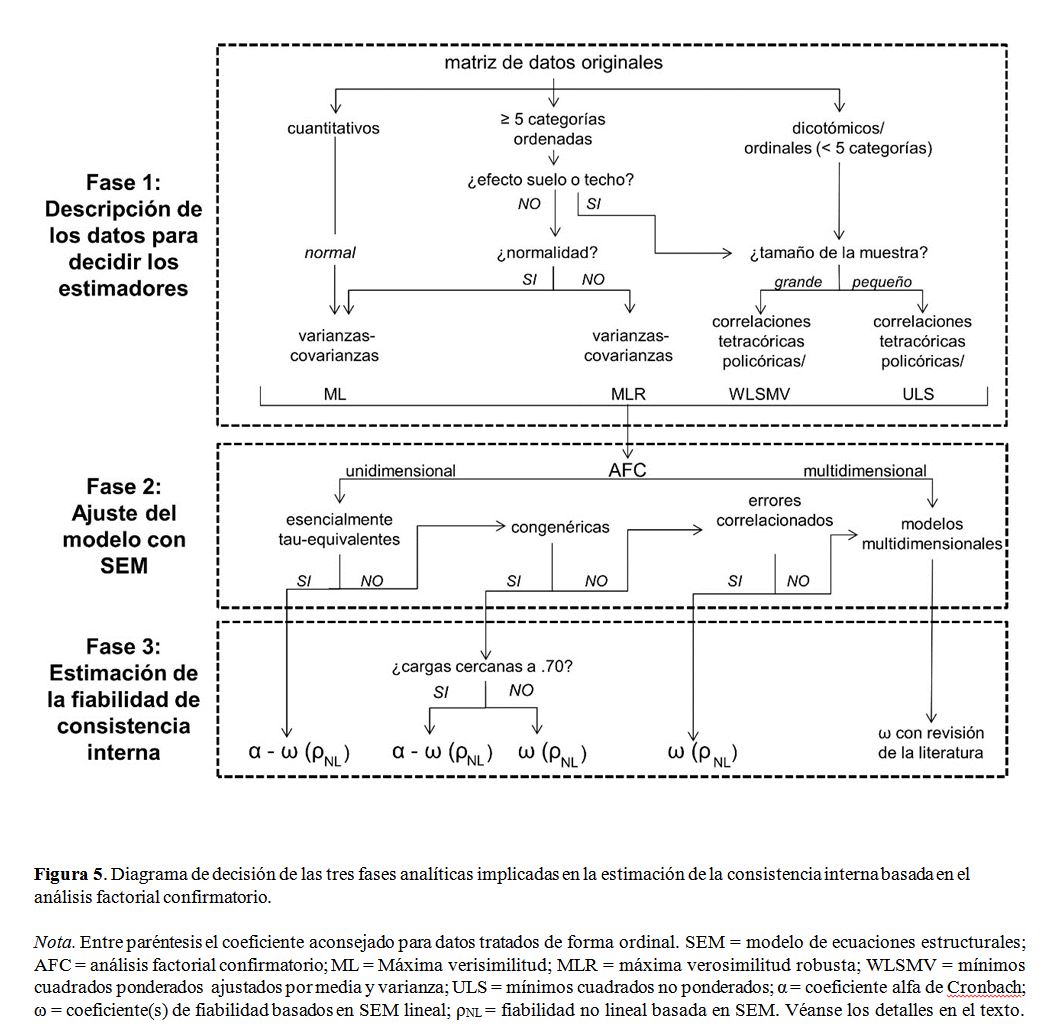
En este trabajo se presenta una guía conceptual y práctica para estimar la fiabilidad de consistencia interna de medidas obtenidas mediante suma o promedio de ítems con base en las aportaciones más recientes de la psicometría. El coeficiente de fiabilidad de consistencia interna se presenta como un subproducto del modelo de medida subyacente en las respuestas a los ítems y se propone su estimación mediante un procedimiento de análisis de los ítems en tres fases, a saber, análisis descriptivo, comprobación de los modelos de medida pertinentes y cálculo del coeficiente de consistencia interna y su intervalo de confianza. Se proporcionan las siguientes fórmulas: (a) los coeficientes alfa de Cronbach y omega para medidas unidimensionales con ítems cuantitativos (b) los coeficientes omega ordinal, alfa ordinal y de fiabilidad no lineal para ítems dicotómicos y ordinales, y (c) los coeficientes omega y omega jerárquico para medidas esencialmente unidimensionales con efectos de método. El procedimiento se generaliza al análisis de medidas obtenidas por suma ponderada, de escalas multidimensionales, de diseños complejos con datos multinivel y/o faltantes y también al desarrollo de escalas. Con fines ilustrativos se expone el análisis de cuatro ejemplos numéricos y se proporcionan los datos y la sintaxis en R.
Descargas
Citas
Abad, F. J., Olea, J., Ponsoda, V., & García, C. (2011). Medición en ciencias sociales y de la salud [Measurement in social and health sciences]. Madrid: Síntesis.
American Psychological Association (2010). Publication manual of the American Psychological Association. (6th ed.). Washington, DC
Behrens, J. T., DiCerbo, K. E., Yel, N., & Levy, R. (2012). Exploratory Data Analysis. In Handbook of Psychology, Second Edition. John Wiley & Sons, Inc. doi:10.1002/9781118133880.hop202002
Bentler, P. M. (2009). Alpha, dimension-free, and model-based internal consistency reliability. Psychometrika, 74(1), 137–143. doi:10.1007/s11336-008-9100-1
Bentler, P. M. (2016). Specificity-enhanced reliability coefficients. Psychological Methods, 0. Advance online publication. doi:10.1037/met0000092
Birnbaum, A. (1968). Some latent trait models and their use in inferring a examinee’s ability. In F. M. Lord & M. R. Novick (Eds.), Statistical Theories of Mental Test Scores (pp. 397–479). Reading, MA: Addison-Wesley.
Black, R. A., Yang, Y., Beitra, D., & McCaffrey, S. (2015). Comparing fit and reliability estimates of a psychological instrument using second-order CFA, bifactor, and essentially tau-equivalent (coefficient alpha) Models via AMOS 22. Journal of Psychoeducational Assessment, 33(5), 451–472. doi:10.1177/0734282914553551
Bovaird, J. A., & Koziol, N. A. (2012). Measurement models for ordered-categorical indicators. In Handbook of Structural Equation Modeling (pp. 495–511). New York, NY: The Guilford Press.
Bollen, K. (1980). Issues in the comparative measurement of political democracy. American Sociological Review, 45, 370–390.
Brown, T. A. (2015). Confirmatory factor analysis for applied research. 2nd Ed. London: The Guilford Press.
Brunner, M., Nagy, G., & Wilhelm, O. (2012). A tutorial on hierarchically structured constructs. Journal of Personality, 80(4), 796–846. doi:10.1111/j.1467-6494.2011.00749.x
Cheng, Y., Yuan, K. H., & Liu, C. (2012). Comparison of reliability measures under factor analysis and item response theory. Educational and Psychological Measurement, 72(1), 52–67. doi:10.1177/0013164411407315
Cho, E. (2016). Making Reliability Reliable: A Systematic approach to reliability coefficients. Organizational Research Methods, 19(4), 651–682. doi:10.1177/1094428116656239
Conway, J. M., & Lance, C. E. (2010). What reviewers should expect from authors regarding common method bias in organizational research. Journal of Business and Psychology, 25(3), 325–334. doi:10.1007/s10869-010-9181-6
Cronbach, L. (1951). Coefficient alpha and the internal structure of tests. Psychometrika, 16(3), 297-334.
Cronbach, L. J., & Shavelson, R. J. (2004). My current thoughts on coefficient alpha and successor procedures. Educational and Psychological Measurement, 64(3), 391–418. doi:10.1177/0013164404266386
Crutzen, R., & Peters, G. (2015). Scale quality: alpha is an inadequate estimate and factor-analytic evidence is needed first of all. Health Psychology Review, 1–6. doi:10.1080/17437199.2015.1124240
Davenport, E. C., Davison, M. L., Liou, P. Y., & Love, Q. U. (2016). Easier said than done: rejoinder on Sijtsma and on Green and Yang. Educational Measurement: Issues and Practice, 35(1), 6–10. doi:10.1111/emip.12106
Deng, L., & Chan, W. (2016). Testing the difference between reliability coefficients alpha and omega. Educational and Psychological Measurement, online, 1–19. doi:10.1177/0013164416658325
Dimitrov, D. M. (2003). Reliability and true-score measures of binary items as a function of their Rasch difficulty parameter. Journal of Applied Measurement, 4(3), 222–233. doi:10.1177/0146621603258786
Dunn, T. J., Baguley, T., & Brunsden, V. (2014). From alpha to omega: a practical solution to the pervasive problem of internal consistency estimation. British Journal of Psychology, 105(3), 399–412. doi:10.1111/bjop.12046
Elosua, P., & Zumbo, B. D. (2008). Reliability coefficients for ordinal response scales. Psicothema, 20(4), 896–901.
Enders, C. K. (2010). Applied missing data analysis. New York, NY: The Guilford Press.
Enders, C. K. (2013). Dealing with missing data in developmental research. Child Development Perspectives, 7(1), 27–31. doi:10.1111/cdep.12008
Ferrando, P. J., & Lorenzo-Seva, U. (2014). El análisis factorial exploratorio de los ítems: Algunas consideraciones adicionales [Exploratory item factor analysis: some additional considerations] Anales de Psicologia, 30(3), 1170–1175. doi:10.6018/analesps.30.3.199991
Ferrando, P. J., & Lorenzo-Seva, U. (2017). Program FACTOR at 10: origins, development and future directions. Psicothema, 29(2), 236–240. https://doi.org/10.7334/psicothema2016.304
Forero, C. G., Maydeu-Olivares, A., & Gallardo-Pujol, D. (2009). Factor analysis with ordinal indicators: A Monte Carlo study comparing DWLS and ULS estimation. Structural Equation Modeling, 16(4), 625–641. doi:10.1080/10705510903203573
Gabler, S., & Raykov, T. (2017). Evaluation of maximal reliability for unidimensional measuring instruments with correlated errors. Structural Equation Modeling: A Multidisciplinary Journal, 24(1), 104-111. Advance online publication. doi:10.1080/10705511.2016.1159916
Gadermann, A. M., Guhn, M., & Zumbo, B. D. (2012). Estimating ordinal reliability for likert-type and ordinal item response data: A conceptual, empirical, and practical guide. Practical Assessment, Research and Evaluation, 17(3), 1–13.
Gignac, G. E. (2014). On the inappropriateness of using items to calculate total scale score reliability via coefficient alpha for multidimensional scales. European Journal of Psychological Assessment, 30(2), 130–139. doi:10.1027/1015-5759/a000181
Graham, J. (2006). Congeneric and (essentially) tau-equivalent estimates of score reliability. What they are and how to use them. Educational and Psychological Measurement, 66(6), 930–944. doi: 10.1177/0013164406288165.
Graham, J. W. (2009). Missing data analysis: Making it work in the real world. Annual Review of Psychology, 60, 549–76. doi:10.1146/annurev.psych.58.110405.085530
Green, S. B., & Yang, Y. (2009). Reliability of summed item scores using structural equation modeling: An alternative to coefficient alpha. Psychometrika, 74(1), 155–167. doi:10.1007/s11336-008-9099-3
Green, S. B., & Yang, Y. (2015). Evaluation of dimensionality in the assessment of internal consistency reliability: coefficient alpha and omega coefficients. Educational Measurement: Issues and Practice, 34(4), 14–20. doi:10.1111/emip.12100
Green, S. B., Yang, Y., Alt, M., Brinkley, S., Gray, S., Hogan, T., & Cowan, N. (2016). Use of internal consistency coefficients for estimating reliability of experimental task scores. Psychonomic Bulletin & Review, 23(3), 750–763. doi:10.3758/s13423-015-0968-3
Gu, F., Little, T. D., & Kingston, N. M. (2013). Misestimation of reliability using coefficient alpha and structural equation modeling when assumptions of tau-equivalence and uncorrelated errors are violated. Methodology, 9(1), 30–40. doi:10.1027/1614-2241/a000052
Hancock, G. R., & Mueller, R. O. (2001). Rethinking construct reliability within latent variable systems. In R. Cudeck, S. du Toit, & D. Sorbom (Eds.), Structural Equation Modeling: Present and future (pp. 195–216). Lincolnwood, IL: Scientific Software International, Inc.
Hancock, G. R., & Mueller, R. O. (Eds.). (2013). Structural equation modeling. A second course (2nd ed.). Charlotte, NC: Information Age Publishing.
Hoyle, R. H. (Ed.). (2012). Handbook of Structural equation modeling. New York, NY: The Guilford Press.
Huggins-Manley, A. C., & Han, H. (2017). Assessing the sensitivity of weighted least squares model fit indexes to local dependence in item response theory models. Structural Equation Modeling: A Multidisciplinary Journal, 24(3), 331–340. doi:10.1080/10705511.2016.1247355
Izquierdo, I., Olea, J., & Abad, F. J. (2014). Exploratory factor analysis in validation studies: Uses and recommendations. Psicothema, 26(3), 395–400. doi:10.7334/psicothema2013.349
Jöreskog, K. G. (1971). Statistical analysis of sets of congeneric tests. Psychometrika, 36(2), 109–133. doi:10.1007/s00170-004-2446-3
Kelley, K., & Pornprasertmanit, S. (2016). Confidence intervals for population reliability coefficients : Evaluation of methods, recommendations, and software for composite measures. Psychological Methods, 21(1), 69–92. doi:10.1037/a0040086
Kim, S., & Feldt, L. S. (2010). The estimation of the IRT reliability coefficient and its lower and upper bounds, with comparisons to CTT reliability statistics. Asia Pacific Education Review, 11(2), 179–188. doi:10.1007/s12564-009-9062-8
Lance, C. E., Dawson, B., Birkelbach, D., & Hoffman, B. J. (2010). Method effects, measurement error, and substantive conclusions. Organizational Research Methods, 13(3), 435–455. doi:10.1177/1094428109352528
Little, T. D., Rhemtulla, M., Gibson, K., & Schoemann, A. M. (2013). Why the items versus parcels controversy needn’t be one. Psychological Methods, 18(3), 285–300. doi:10.1037/a0033266
Lloret-Segura, S., Ferreres-Traver, A., Hernández-Baeza, A., & Tomás-Marco, I. (2014). El análisis factorial exploratorio de los ítems: una guía práctica, revisada y actualizada. [Exploratory item factor analysis: A practical guide revised and up-dated] Anales de Psicología, 30(3), 1151–1169. doi:10.6018/analesps.30.3.199361
Lord, F. M., & Novick, M. R. (1968). Statistical theories of mental test scores. Reading, MA: Addison Wesley.
Malone, P. S., & Lubansky, J. B. (2012). Preparing data for structural equation modeling: doing your homework. In R. H. Hoyle (Ed.), Handbook of Structural Equation Modeling (pp. 263–276). New York, NY: The Guilford Press.
Mansolf, M., & Reise, S. P. (2016). Exploratory bifactor analysis: The Schmid-Leiman orthogonalization and Jennrich-Bentler analytic rotations. Multivariate Behavioral Research, 51(5), 698–717. doi:10.1080/00273171.2016.1215898
Marsh, H. W. (1996). Positive and negative global self-esteem: a substantively meaningful distinction or artifactors? Journal of Personality and Social Psychology, 70(4), 810–819. doi:10.1037/0022-3514.70.4.810
Marsh, H. W., Lüdtke, O., Muthén, B., Asparouhov, T., Morin, A. J. S., Trautwein, U., & Nagengast, B. (2010). A new look at the big five factor structure through exploratory structural equation modeling. Psychological Assessment, 22(3), 471–91. doi:10.1037/a0019227
Marsh, H. W., Lüdtke, O., Nagengast, B., Morin, A. J. S., & Von Davier, M. (2013). Why item parcels are (almost) never appropriate: Two wrongs do not make a right--camouflaging misspecification with item parcels in CFA models. Psychological Methods, 18, 257–84. doi:10.1037/a0032773
Maydeu-Olivares, A., & Coffman, D. L. (2006). Random intercept item factor analysis. Psychological Methods, 11(4), 344–362. doi:10.1037/1082-989X.11.4.344
Maydeu-Olivares, A., Fairchild, A. J., & Hall, A. G. (2017). Goodness of fit in item factor analysis: effect of the number of response alternatives. Structural Equation Modeling: A Multidisciplinary Journal, 0(0), 1–11. doi:10.1080/10705511.2017.1289816
McCrae, R. R. (2014). A more nuanced view of reliability: specificity in the trait hierarchy. Personality and Social Psychology Review, 19(2), 97–112. doi:10.1177/1088868314541857
McDonald, R. P. (1999). Test theory: A unified treatment. Mahwah, NJ: Lawrence Erlbaum Associates.
McNeish, D. (2017). Thanks coefficient alpha, we’ll take it from here. Psychological Methods, 0. Advance online publication. doi: 10.1037/met0000144
Muñiz, J. (1992). Teoría clásica de los tests [Classical test theory]. Madrid: Pirámide.
Muthén, B. (1984). A general structural equation model with dichotomous, ordered categorical, and continuous latent variable indicators. Psychometrika, 49(1), 115–132.
Muthén, L. K., & Muthén, B. O. (2017). Mplus User’s Guide (Eighth Edition). Los Angeles, CA: Muthén & Muthén.
Muthén & Muthén (n.d.). Chi-Square difference testing using the Satorra-Bentler scaled Chi-Square. Retrieved June 19, 2017 from https://www.statmodel.com/chidiff.shtml
Napolitano, C. M., Callina, K. S., & Mueller, M. K. (2013). Comparing alternate approaches to calculating reliability for dichotomous data: The sample case of adolescent selection, optimization, and compensation. Applied Developmental Science, 17(3), 148–151. doi:10.1080/10888691.2013.804372
Ntoumanis, N., Mouratidis, T., Ng, J. Y. Y., & Viladrich, C. (2015). Advances in quantitative analyses and their implications for sport and exercise psychology research. In S. Hanton & S. D. Mellalieu (Eds.), Contemporary advances in sport psychology: A review. (pp. 226–257). London: Routledge.
Nunnally, J. C. (1978). Psychometric theory. New York, NY: McGrawHill.
Padilla, M. A., & Divers, J. (2016). A comparison of composite reliability estimators: coefficient omega confidence intervals in the current literature. Educational and Psychological Measurement, 76(3), 436–453. doi:10.1177/0013164415593776
R Core Team (2016). R: A language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria. Retrived from https://www.R-project.org/.
Raykov, T. (1997). Estimation of composite reliability for congeneric measures. Applied Psychological Measurement, 21(2), 173–184. doi: 0803973233
Raykov, T. (1998). Coefficient alpha and composite reliability with interrelated nonhomogeneous items. Applied Psychological Measurement, 22(4), 375–385. doi:10.1177/014662169802200407
Raykov, T. (2001). Bias of coefficient alpha for fixed congeneric measures with correlated errors. Applied Psychological Measurement, 25(1), 69–76. doi:10.1177/01466216010251005
Raykov, T. (2004). Point and interval estimation of reliability for multiple-component measuring instruments via linear constraint covariance structure modeling. Structural Equation Modeling, 11(3), 452–483. doi:10.1207/s15328007sem1103
Raykov, T. (2007). Reliability if deleted, not “alpha if deleted”: Evaluation of scale reliability following component deletion. The British Journal of Mathematical and Statistical Psychology, 60(2), 201–216. doi: 10.1348/000711006X115954
Raykov, T. (2012). Scale construction and development using structural equation modeling. In R. H. Hoyle (Ed.), Handbook of Structural Equation Modeling (pp. 472–492). New York, NY: Guilford Press.
Raykov, T., Dimitrov, D. M., & Asparouhov, T. (2010). Evaluation of scale reliability with binary measures using latent variable modeling. Structural Equation Modeling: A Multidisciplinary Journal, 17(2), 265–279. doi:10.1080/10705511003659417
Raykov, T., Gabler, S., & Dimitrov, D. M. (2016). Maximal reliability and composite reliability: examining their difference for multicomponent measuring instruments using latent variable modeling. Structural Equation Modeling: A Multidisciplinary Journal, 23(3), 384–391. doi:10.1080/10705511.2014.966369
Raykov, T., & Marcoulides, G. A. (2012). Evaluation of validity and reliability for hierarchical scales using latent variable modeling. Structural Equation Modeling: A Multidisciplinary Journal, 19(3), 495–508. doi:10.1080/10705511.2012.687675
Raykov, T., & Marcoulides, G. A. (2014). Scale reliability evaluation with heterogeneous populations. Educational and Psychological Measurement, 75(5), 875–892. doi:10.1177/0013164414558587
Raykov, T., & Marcoulides, G. A. (2015). A direct latent variable modeling based method for point and interval estimation of coefficient alpha. Educational and Psychological Measurement, 75(1), 146–156. doi:10.1177/0013164414526039
Raykov, T., & Marcoulides, G. A. (2016a). Scale reliability evaluation under multiple assumption violations. Structural Equation Modeling: A Multidisciplinary Journal, 23(2), 302–313. doi:10.1080/10705511.2014.938597
Raykov, T., & Marcoulides, G. A. (2016b). On Examining specificity in latent construct indicators. Structural Equation Modeling: A Multidisciplinary Journal, 23(6), 845-855. doi:10.1080/10705511.2016.1175947
Raykov, T., & Pohl, S. (2013). Essential unidimensionality examination for multicomponent scales: an interrelationship decomposition approach. Educational and Psychological Measurement, 73(4), 581–600. doi:10.1177/0013164412470451
Raykov, T., West, B. T., & Traynor, A. (2015). Evaluation of coefficient alpha for multiple-component measuring instruments in complex sample designs. Structural Equation Modeling: A Multidisciplinary Journal, 22(3), 429–438. doi:10.1080/10705511.2014.936081
Reise, S. P. (2012). The rediscovery of bifactor measurement models. Multivariate Behavioral Research, 47(5), 667–696. doi:10.1080/00273171.2012.715555
Revelle, W. (2016). psych: Procedures for personality and psychological research. R package version 1.6.4. North-western University, Evanston. Retrieved June 19, 2017 from http://cran.r-project.org/web/packages/psych/.
Revelle, W., & Zinbarg, R. E. (2009). Coefficient alpha, beta, omega, and the GLB: Comment on Sitjsma. Psychometrika, 74(1), 145–154.
Rhemtulla, M., Brosseau-Liard, P. É., & Savalei, V. (2012). When can categorical variables be treated as continuous? A comparison of robust continuous and categorical SEM estimation methods under suboptimal conditions. Psychological Methods, 17(3), 354–373. doi:10.1037/a0029315
Rodriguez, A., Reise, S. P., & Haviland, M. G. (2016). Evaluating bifactor models: Calculating and interpreting statistical indices. Psychological Methods, 21(2), 137–150. doi:10.1037/met0000045
Rosseel, Y. (2012). lavaan: An R Package for structural equation modeling. Journal of Statistical Software, 48(2), 1–36.
Sass, D. a., Schmitt, T. a., & Marsh, H. W. (2014). Evaluating model fit with ordered categorical data within a measurement invariance framework: A comparison of estimators. Structural Equation Modeling: A Multidisciplinary Journal, 21(2), 167–180. doi:10.1080/10705511.2014.882658
semTools Contributors. (2016). semTools: Useful tools for structural equation modeling. R package version 0-4-11.
Sijtsma, K. (2009). On the use, the misuse, and the very limited usefulness of Cronbach’s alpha. Psychometrika, 74(1), 107–120. doi:10.1007/s11336-008-9101-0
Sijtsma, K. (2015). Delimiting coefficient alpha from internal consistency and unidimensionality. Educational Measurement: Issues and Practice, 34(4), 10–13.
Spector, P. E. (2006). Method variance in organiztional research. Truth or urban legend ? Organizational Research Methods, 9(2), 221–232. doi:1094428105284955
Stout, W. (1987). A nonparametric approach for assessing latent trait unidimensionality. Psychometrika, 52(4), 589–617. doi:10.1007/BF02294821
Wickham, H. (2007). Reshaping data with the reshape package. Journal of Statistical Software, 21(12), 1-20. Retrieved from http://www.jstatsoft.org/v21/i12/.
Yang, Y., & Green, S. B. (2010). A note on structural equation modeling estimates of reliability. Structural Equation Modeling: A Multidisciplinary Journal, 17(1), 66–81. doi:10.1080/10705510903438963
Yang, Y., & Green, S. B. (2011). Coefficient alpha: A reliability coefficient for the 21st Century? Journal of Psychoeducational Assessment, 29(4), 377–392. doi:10.1177/0734282911406668
Yang, Y., & Green, S. B. (2015). Evaluation of structural equation modeling estimates of reliability for scales with ordered categorical items. Methodology, 11(1), 23–34. doi:10.1027/1614-2241/a000087
Zhang, Z., & Yuan, K.-H. (2016). Robust coefficients alpha and omega and confidence intervals with outlying observations and missing data: Methods and software. Educational and Psychological Measurement, 76(3), 387–411. doi:10.1177/0013164415594658
Zinbarg, R. E., Revelle, W., Yovel, I., & Li, W. (2005). Cronbach’s α, Revelle’s β, and Mcdonald’s ωH: their relations with each other and two alternative conceptualizations of reliability. Psychometrika, 70(1), 123–133. doi:10.1007/s11336-003-0974-7
Zumbo, B. D., Gadermann, A. M., & Zeisser, C. (2007). Ordinal versions of coefficients alpha and theta for Likert rating scales. Journal of Modern Applied Statistical Methods, 6(1), 21–29. doi:10.1107/S0907444909031205
Las obras que se publican en esta revista están sujetas a los siguientes términos:
1. El Servicio de Publicaciones de la Universidad de Murcia (la editorial) conserva los derechos patrimoniales (copyright) de las obras publicadas, y favorece y permite la reutilización de las mismas bajo la licencia de uso indicada en el punto 2.
© Servicio de Publicaciones, Universidad de Murcia, 2024
2. Las obras se publican en la edición electrónica de la revista bajo una licencia Creative Commons Reconocimiento-CompartirIgual 4.0 Internacional (texto legal). Se pueden copiar, usar, difundir, transmitir y exponer públicamente, siempre que: i) se cite la autoría y la fuente original de su publicación (revista, editorial y URL de la obra); ii) no se usen para fines comerciales; iii) se mencione la existencia y especificaciones de esta licencia de uso.
3. Condiciones de auto-archivo. Se permite y se anima a los autores a difundir electrónicamente las versiones pre-print (versión antes de ser evaluada y enviada a la revista) y/o post-print (versión evaluada y aceptada para su publicación) de sus obras antes de su publicación, ya que favorece su circulación y difusión más temprana y con ello un posible aumento en su citación y alcance entre la comunidad académica. Color RoMEO: verde.